Acceleration - Non-uniform Circular Motion Calculator

Radial Acceleration (a_rad):

Tangential Acceleration (a_tan):

Total Acceleration (m/s²): Total Acceleration (ft/s²): Total Acceleration (cm/s²): Total Acceleration (km/h²): Total Acceleration (mph/s²): Total Acceleration (g):

1. What is Total Acceleration in Non-uniform Circular Motion?

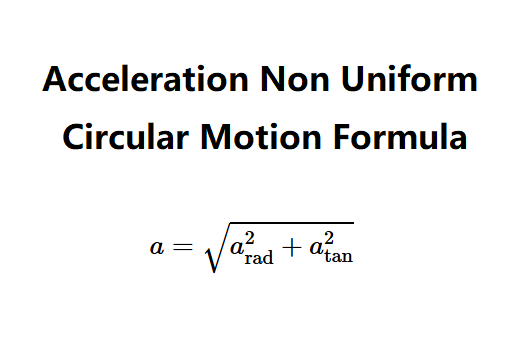
Definition: The total acceleration in non-uniform circular motion is the vector sum of radial (centripetal) and tangential accelerations, calculated using the formula:

\[ a = \sqrt{a_{\text{rad}}^2 + a_{\text{tan}}^2} \]

Variables:

\( a \): Total acceleration (in m/s², convertible to ft/s², cm/s², km/h², mph/s², g).
\( a_{\text{rad}} \): Radial (centripetal) acceleration (convertible to m/s²).
\( a_{\text{tan}} \): Tangential acceleration (convertible to m/s²).

Explanation: In non-uniform circular motion, radial acceleration keeps the object in a circular path, while tangential acceleration changes its speed.

2. Importance of Total Acceleration

Details: Total acceleration is crucial in physics and engineering for analyzing motion in circular paths, such as in vehicles, amusement rides, or orbital mechanics.

3. Using the Calculator

Tips: Enter the radial and tangential accelerations in m/s² or other supported units. Click "Calculate" to get the total acceleration in multiple units (m/s², ft/s², cm/s², km/h², mph/s², g). Results greater than 10,000 or less than 0.00001 will be displayed in scientific notation.

Frequently Asked Questions (FAQ)

Q1: What is non-uniform circular motion?
A: It is circular motion where the speed of the object changes, involving both radial and tangential accelerations.

Q2: What is radial acceleration?
A: Radial (centripetal) acceleration keeps an object moving in a circular path, directed toward the center.

Q3: What is tangential acceleration?
A: Tangential acceleration changes the speed of the object along the circular path, tangent to the trajectory.

Q4: What units are used?
A: Inputs can be in m/s², ft/s², cm/s², km/h², mph/s², or g. The output is in m/s², convertible to other units.

Q5: How accurate is this calculator?
A: The calculator is accurate based on the formula, assuming the input accelerations are correct.

Favorite