Angular Deflection of a Torsion Solid Shaft Calculator

Twisting Moment (T):

Length of Shaft (L):

Modulus of Rigidity (G):

Shaft Outside Diameter (D):

Angular Deflection (θ) in radians: Angular Deflection (θ) in degrees:

1. What is Angular Deflection of a Torsion Solid Shaft?

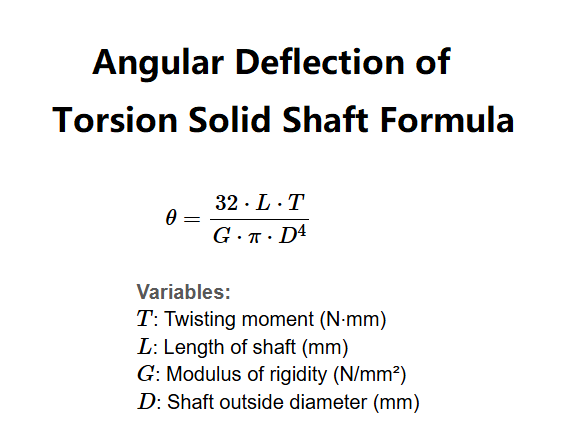
Definition: Angular deflection (θ) is the angle of twist experienced by a solid shaft when subjected to a twisting moment (T).

Purpose: It is used in mechanical engineering to determine the torsional stiffness and deformation of shafts under load.

2. How Does the Calculator Work?

The angular deflection is calculated using the formula:

\[ \theta = \frac{32 \cdot L \cdot T}{G \cdot \pi \cdot D^4} \]

Variables:

\( T \): Twisting moment (N⋅mm)
\( L \): Length of shaft (mm)
\( G \): Modulus of rigidity (N/mm²)
\( D \): Shaft outside diameter (mm)

Explanation: The formula calculates the angular deflection in radians. The result can also be converted to degrees for easier interpretation.

3. Importance of Angular Deflection Calculation

Details: This calculation is essential for designing shafts in machinery, ensuring they can withstand torsional forces without excessive deformation.

4. Using the Calculator

Tips: Enter the twisting moment, length of the shaft, modulus of rigidity, and shaft diameter. Click "Calculate" to get the angular deflection in radians and degrees.

Frequently Asked Questions (FAQ)

Q1: What is the significance of the modulus of rigidity (G)?
A: The modulus of rigidity (G) measures a material's resistance to shear stress. It is crucial for determining how much a shaft will twist under a given load.

Q2: Can I use this calculator for hollow shafts?
A: No, this calculator is designed specifically for solid shafts. For hollow shafts, a different formula is required.

Q3: Why is the result displayed in both radians and degrees?
A: Radians are the standard unit for angular measurements in physics and engineering, but degrees are more intuitive for many users. Displaying both ensures clarity.

Q4: What happens if I input zero for any value?
A: The calculator requires all inputs to be positive numbers. If any value is zero or negative, the calculation will not proceed.

Q5: How accurate is this calculator?
A: The calculator provides accurate results based on the formula and input values. However, real-world conditions may introduce additional factors not accounted for here.

Favorite