Constant Acceleration Calculator

Initial Velocity (\( V_i \)):

Final Velocity (\( V_f \)):

Time (\( t \)):

Acceleration (\( a \)) in meters/second² (m/s²):

Acceleration (\( a \)) in feet/second² (ft/s²):

Acceleration (\( a \)) in g:

1. What is the Constant Acceleration Calculator?

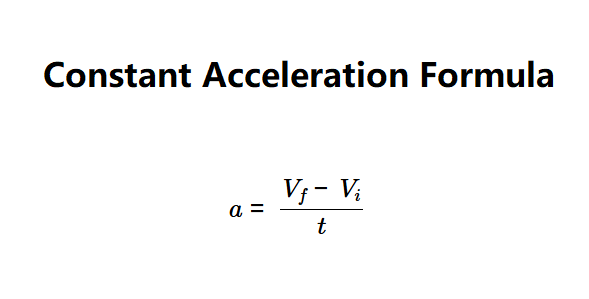
Definition: This calculator determines the constant acceleration (\( a \)) of an object, based on its initial velocity (\( V_i \)), final velocity (\( V_f \)), and time (\( t \)).

Purpose: It assists in understanding the rate of change of velocity over time, useful in physics, engineering, and automotive applications such as motion analysis and vehicle performance.

2. How Does the Calculator Work?

The calculator uses the relationship:

\[ a = \frac{V_f - V_i}{t} \]

Where:

\( a \) — Acceleration (in various units)
\( V_f \) — Final velocity
\( V_i \) — Initial velocity
\( t \) — Time of motion

Explanation: Enter the initial velocity, final velocity, and time in the chosen units, and the calculator computes the acceleration. Results use scientific notation (5 decimal places) if the acceleration in m/s² is greater than 10000 or less than 0.00001, otherwise 2 decimal places. For default inputs (\( V_i = 5 \, \text{m/s} \), \( V_f = 15 \, \text{m/s} \), \( t = 2 \, \text{s} \)), the calculated acceleration is 5 m/s².

3. Importance of Acceleration

Details: Acceleration measures the rate of change of velocity, indicating how quickly an object’s speed or direction changes. It’s essential for analyzing motion in vehicles, machinery, and projectile trajectories.

4. Using the Calculator

Tips: Enter positive or negative values for initial and final velocities (depending on direction), a positive value for time, and ensure \( t \neq 0 \), then click "Calculate." Results show the acceleration in meters/second², feet/second², and g (scientific notation with 5 decimal places if > 10000 or < 0.00001 m/s², otherwise 2 decimal places).

Favorite