Continuity Equation (area) Calculator

Area of Second Tube (\( A_2 \)):

Velocity in First Tube (\( v_1 \)):

Velocity in Second Tube (\( v_2 \)):

Density in First Tube (\( \rho_1 \)):

Density in Second Tube (\( \rho_2 \)):

Area of First Tube (\( A_1 \)) in meters² (m²):

Area of First Tube (\( A_1 \)) in centimeters² (cm²):

Area of First Tube (\( A_1 \)) in feet² (ft²):

1. What is the Continuity Equation Calculator?

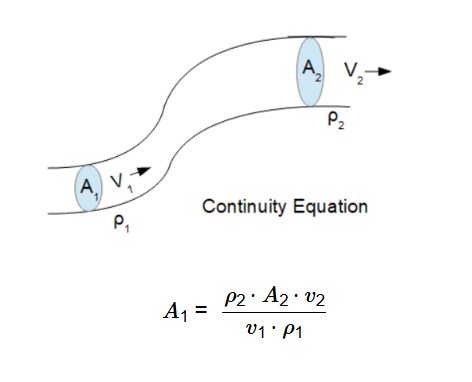
Definition: This calculator computes the cross-sectional area of the first tube (\( A_1 \)) in a fluid flow system, based on the continuity equation for mass conservation, considering the area (\( A_2 \)), velocities (\( v_1 \), \( v_2 \)), and densities (\( \rho_1 \), \( \rho_2 \)) of the fluid in two sections of a tube.

Purpose: It is used in fluid dynamics to analyze flow in pipes, ducts, or channels, applicable in engineering fields like aerodynamics, hydraulics, and HVAC systems.

2. How Does the Calculator Work?

The calculator uses the relationship derived from the continuity equation:

\[ A_1 = \frac{\rho_2 \cdot A_2 \cdot v_2}{v_1 \cdot \rho_1} \]

Where:

\( A_1 \) — Cross-sectional area of the first tube (in various units)
\( A_2 \) — Cross-sectional area of the second tube
\( v_1 \) — Velocity of the fluid in the first tube
\( v_2 \) — Velocity of the fluid in the second tube
\( \rho_1 \) — Density of the fluid in the first tube
\( \rho_2 \) — Density of the fluid in the second tube

Explanation: Enter the area of the second tube, velocities, and densities in the chosen units, and the calculator computes the area of the first tube (\( A_1 \)). Results are displayed with 5 decimal places. For default inputs (\( A_2 = 0.05 \, \text{m}^2 \), \( v_1 = 2 \, \text{m/s} \), \( v_2 = 5 \, \text{m/s} \), \( \rho_1 = 1.225 \, \text{kg/m}^3 \), \( \rho_2 = 1.225 \, \text{kg/m}^3 \)), the calculated area \( A_1 \) is 0.12500 m².

3. Importance of the Continuity Equation

Details: The continuity equation ensures mass conservation in fluid flow, which is crucial for designing systems involving fluid transport, such as pipelines, ventilation systems, and aircraft wings.

4. Using the Calculator

Tips: Enter positive values for all inputs with up to 4 decimal places (step of 0.0001), ensure \( v_1 \neq 0 \) and \( \rho_1 \neq 0 \), then click "Calculate." Results show the area \( A_1 \) in meters², centimeters², and feet², always with 5 decimal places.

Favorite