Distance Traveled Calculator

Initial Velocity (\( v_0 \)):

End Time (\( t_1 \)):

Initial Time (\( t_0 \)):

Initial Position (\( s_0 \)):

Constant Acceleration (\( a \)):

Distance Traveled (\( s(t) \)) in meters (m):

Distance Traveled (\( s(t) \)) in kilometers (km):

Distance Traveled (\( s(t) \)) in miles (mi):

Distance Traveled (\( s(t) \)) in feet (ft):

Distance Traveled (\( s(t) \)) in yards (yd):

1. What is the Distance Traveled Calculator?

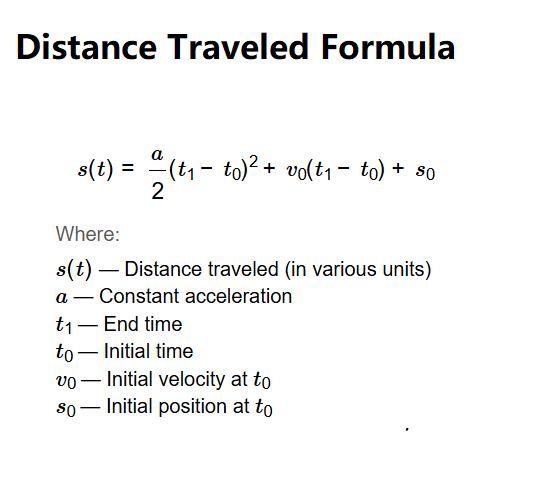
Definition: This calculator computes the distance traveled (\( s(t) \)) by an object under constant acceleration, based on its initial velocity (\( v_0 \)), initial time (\( t_0 \)), end time (\( t_1 \)), initial position (\( s_0 \)), and constant acceleration (\( a \)).

Purpose: It is used in physics and engineering to analyze motion with constant acceleration, applicable in scenarios like vehicle motion, projectile motion, and mechanical systems.

2. How Does the Calculator Work?

The calculator uses the relationship:

\[ s(t) = \frac{a}{2} (t_1 - t_0)^2 + v_0 (t_1 - t_0) + s_0 \]

Where:

\( s(t) \) — Distance traveled (in various units)
\( a \) — Constant acceleration
\( t_1 \) — End time
\( t_0 \) — Initial time
\( v_0 \) — Initial velocity at \( t_0 \)
\( s_0 \) — Initial position at \( t_0 \)

Explanation: Enter the initial velocity, end time, initial time, initial position, and constant acceleration in the chosen units, and the calculator computes the distance traveled. Results are displayed with 5 decimal places. For default inputs (\( v_0 = 5 \, \text{m/s} \), \( t_1 = 2 \, \text{s} \), \( t_0 = 0 \, \text{s} \), \( s_0 = 0 \, \text{m} \), \( a = 2 \, \text{m/s}^2 \)), the calculated distance \( s(t) \) is approximately 14.00000 meters.

3. Importance of Distance Traveled

Details: Calculating the distance traveled under constant acceleration is crucial for understanding motion dynamics, such as in automotive engineering, aerospace, and physics experiments involving uniform acceleration.

4. Using the Calculator

Tips: Enter values for initial velocity, times, initial position, and acceleration with up to 4 decimal places (step of 0.0001), then click "Calculate." Results show the distance traveled \( s(t) \) in meters, kilometers, miles, feet, and yards, always with 5 decimal places.

Favorite