Harmonic Oscillator - Amplitude Calculator

Initial Displacement (\( x_0 \)):

Initial Velocity (\( v_{0x} \)):

Angular Velocity (\( \omega \)):

Amplitude (\( A \)) in meters (m):

Amplitude (\( A \)) in centimeters (cm):

Amplitude (\( A \)) in inches (in):

1. What is the Harmonic Oscillator - Amplitude Calculator?

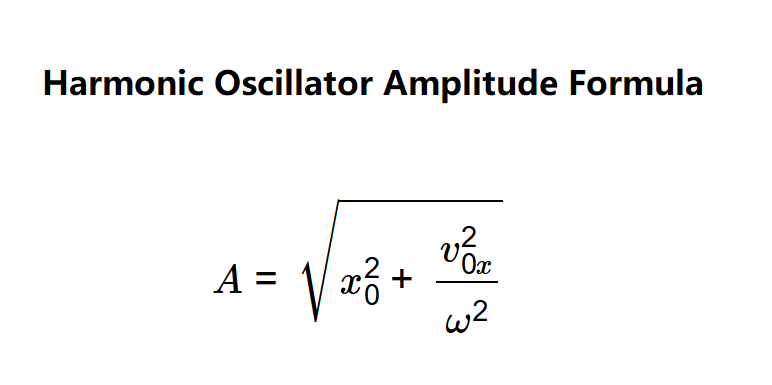
Definition: This calculator computes the amplitude (\( A \)) of a harmonic oscillator based on its initial displacement (\( x_0 \)), initial velocity (\( v_{0x} \)), and angular velocity (\( \omega \)).

Purpose: It is used in physics to determine the maximum displacement of an oscillating system, applicable in mechanics, wave motion, and systems like springs or pendulums.

2. How Does the Calculator Work?

The calculator uses the relationship:

\[ A = \sqrt{x_0^2 + \frac{v_{0x}^2}{\omega^2}} \]

Where:

\( A \) — Amplitude (in various units)
\( x_0 \) — Initial displacement
\( v_{0x} \) — Initial velocity
\( \omega \) — Angular velocity (angular frequency)

Explanation: Enter the initial displacement, initial velocity, and angular velocity in the chosen units, and the calculator computes the amplitude. Results are displayed with 5 decimal places, using scientific notation if the value exceeds 100,000 or is less than 0.0001. For default inputs (\( x_0 = 0.1 \, \text{m} \), \( v_{0x} = 0.2 \, \text{m/s} \), \( \omega = 2 \, \text{rad/s} \)), the calculated amplitude \( A \) is approximately 0.14142 meters.

3. Importance of Harmonic Oscillator Amplitude Calculation

Details: Calculating the amplitude of a harmonic oscillator is essential for understanding the behavior of oscillating systems, such as in mechanical vibrations, electrical circuits, and wave mechanics, aiding in system design and analysis.

FAQ

How do I find the amplitude of a harmonic oscillator?

Measure the initial displacement in meters, the initial velocity in meters/second, and the angular velocity in radians/second. Compute the amplitude using the formula \( A = \sqrt{x_0^2 + \frac{v_{0x}^2}{\omega^2}} \). You will then have the amplitude in meters.

How can I find angular velocity with amplitude and other parameters?

Measure the amplitude in meters, initial displacement in meters, and initial velocity in meters/second. Use the equation \( A = \sqrt{x_0^2 + \frac{v_{0x}^2}{\omega^2}} \) and solve for \( \omega \): \( \omega = \sqrt{\frac{v_{0x}^2}{A^2 - x_0^2}} \), ensuring \( A^2 > x_0^2 \).

What is the formula for the amplitude of a harmonic oscillator?

The formula for the amplitude of a harmonic oscillator is \( A = \sqrt{x_0^2 + \frac{v_{0x}^2}{\omega^2}} \), where \( A \) is the amplitude, \( x_0 \) is the initial displacement, \( v_{0x} \) is the initial velocity, and \( \omega \) is the angular velocity. The standard unit for amplitude is meters (m).

Favorite