Radial Acceleration (R, T) Calculator

Orbital Period (\( T \)):

Orbital Radius (\( r \)):

Radial Acceleration (\( a_{rad} \)) in meters/second² (m/s²):

Radial Acceleration (\( a_{rad} \)) in centimeters/second² (cm/s²):

Radial Acceleration (\( a_{rad} \)) in feet/second² (ft/s²):

1. What is the Radial Acceleration (R, T) Calculator?

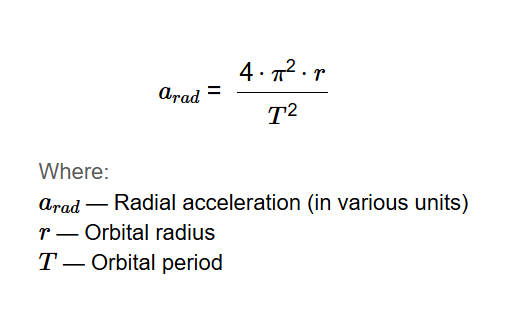
Definition: This calculator computes the radial acceleration (\( a_{rad} \)) of an orbiting body based on its orbital radius (\( r \)) and orbital period (\( T \)).

Purpose: It is used in physics and astronomy to determine the centripetal acceleration required for an object to maintain a circular orbit, applicable in planetary motion and satellite dynamics.

2. How Does the Calculator Work?

The calculator uses the relationship:

\[ a_{rad} = \frac{4 \cdot \pi^2 \cdot r}{T^2} \]

Where:

\( a_{rad} \) — Radial acceleration (in various units)
\( r \) — Orbital radius
\( T \) — Orbital period

Explanation: Enter the orbital period and orbital radius in the chosen units, and the calculator computes the radial acceleration. Results are displayed with 5 decimal places, using scientific notation if the value exceeds 100,000 or is less than 0.0001. For default inputs (\( T = 365.25 \, \text{days} \), \( r = 1 \, \text{au} \)), which approximates Earth’s orbit around the Sun, the calculated radial acceleration \( a_{rad} \) is approximately \(0.00593 \, \text{m/s}^2\).

3. Importance of Radial Acceleration Calculation

Details: Calculating radial acceleration is essential for understanding the dynamics of orbiting bodies, aiding in the design of satellites, space missions, and the study of celestial mechanics.

FAQ

How do I find the radial acceleration of an orbiting body?

Measure the orbital period in seconds and the orbital radius in meters. Compute the radial acceleration using the formula \( a_{rad} = -\frac{4 \cdot \pi^2 \cdot r}{T^2} \). The result will be in meters/second².

Why is the radial acceleration negative?

The negative sign indicates that the acceleration is directed inward toward the center of the orbit, as it is the centripetal acceleration required to keep the body in circular motion.

What is the formula for radial acceleration in terms of radius and period?

The formula for radial acceleration is \( a_{rad} = -\frac{4 \cdot \pi^2 \cdot r}{T^2} \), where \( r \) is the orbital radius, and \( T \) is the orbital period. The standard unit for acceleration is meters/second² (m/s²).

Favorite