Snell's Law (solved for n) Calculator

Calculate:

Refractive Index 1 (n₁):

Refractive Index 2 (n₂):

Angle of Incidence (θi):

Angle of Transmission (θt):

1. What is Snell's Law for Refractive Indices?

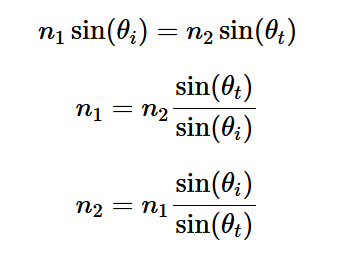
Definition: Snell's Law relates the angles of incidence and refraction (transmission) when light passes between two media with different refractive indices, allowing calculation of either refractive index:

\[ n_1 \sin(\theta_i) = n_2 \sin(\theta_t) \] \[ n_1 = n_2 \frac{\sin(\theta_t)}{\sin(\theta_i)} \] \[ n_2 = n_1 \frac{\sin(\theta_i)}{\sin(\theta_t)} \]

Variables:

\( n_1 \): Refractive index of material 1 (where the light is coming from, dimensionless).
\( n_2 \): Refractive index of material 2 (where the light is entering, dimensionless).
\( \theta_i \): Angle of incidence (in degrees or radians).
\( \theta_t \): Angle of transmission (in degrees or radians).

Explanation: These equations allow you to determine the refractive index of one medium given the refractive index of the other medium and the angles of incidence and transmission.

2. Importance of Snell's Law

Details: Snell's Law is fundamental in optics and physics for understanding and predicting the behavior of light as it travels between different media, used in designing lenses, prisms, and optical devices.

3. Using the Calculator

Tips: Select whether to calculate \( n_1 \) or \( n_2 \), then enter the known refractive index, the angle of incidence, and the angle of transmission with their units (degrees or radians). The input field for the refractive index being calculated will be disabled (grayed out). Click "Calculate" to get the unknown refractive index.

Frequently Asked Questions (FAQ)

Q1: What is Snell's Law?
A: Snell's Law describes the relationship between the angles of incidence and refraction when light passes between two media with different refractive indices.

Q2: What is the refractive index?
A: The refractive index (\( n \)) is a dimensionless number that describes how much light slows down and bends in a medium compared to a vacuum.

Q3: What is the angle of incidence?
A: The angle of incidence (\( \theta_i \)) is the angle between the incoming light ray and the normal (perpendicular) to the surface at the point of incidence.

Q4: What is the angle of transmission?
A: The angle of transmission (\( \theta_t \)) is the angle between the refracted light ray and the normal (perpendicular) to the surface at the point of refraction.

Q5: How accurate is this calculator?
A: The calculator is accurate based on Snell's Law and the input values, assuming ideal conditions. Real-world factors like dispersion or surface imperfections may introduce variations.

Favorite