Tangential Acceleration about Center of Mass Calculator

Radius (\( r \)):

Angular Acceleration (\( \alpha \)):

Tangential Acceleration (\( a_{tan} \)) in meters/second² (m/s²):

Tangential Acceleration (\( a_{tan} \)) in centimeters/second² (cm/s²):

Tangential Acceleration (\( a_{tan} \)) in feet/second² (ft/s²):

1. What is the Tangential Acceleration about Center of Mass Calculator?

Definition: This calculator computes the tangential acceleration (\( a_{tan} \)) of a point mass rotating about the center of mass based on its radius (\( r \)) and angular acceleration (\( \alpha \)).

Purpose: It is used in physics to determine the tangential component of acceleration for rotating bodies, applicable in mechanics, rotational dynamics, and engineering.

2. How Does the Calculator Work?

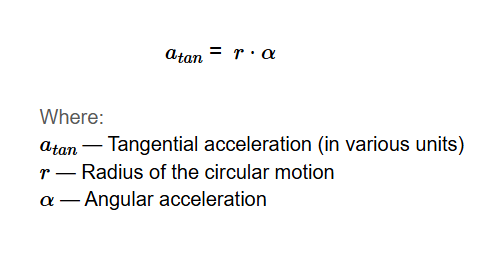
The calculator uses the relationship:

\[ a_{tan} = r \cdot \alpha \]

Where:

\( a_{tan} \) — Tangential acceleration (in various units)
\( r \) — Radius of the circular motion
\( \alpha \) — Angular acceleration

Explanation: Enter the radius and angular acceleration in the chosen units, and the calculator computes the tangential acceleration. Results are displayed with 5 decimal places, using scientific notation if the value exceeds 100,000 or is less than 0.0001. For default inputs (\( r = 1 \, \text{m} \), \( \alpha = 2 \, \text{rad/s}^2 \)), the calculated tangential acceleration \( a_{tan} \) is 2.00000 m/s².

3. Importance of Tangential Acceleration Calculation

Details: Calculating tangential acceleration is essential for understanding the linear acceleration component due to angular changes, aiding in the analysis of rotating machinery, planetary motion, and vehicle dynamics.

FAQ

How do I find the tangential acceleration?

Measure the radius in meters and the angular acceleration in radians/second². Compute the tangential acceleration using the formula \( a_{tan} = r \cdot \alpha \). The result will be in meters/second².

What does tangential acceleration represent?

Tangential acceleration represents the rate of change of the tangential speed of a point mass in circular motion, caused by the angular acceleration of the rotating body.

What is the formula for tangential acceleration?

The formula for tangential acceleration is \( a_{tan} = r \cdot \alpha \), where \( r \) is the radius, and \( \alpha \) is the angular acceleration. The standard unit for acceleration is meters/second² (m/s²).

Favorite